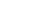What three numbers multiply to get 532? In other words, you want to know all the combinations of 3 numbers that can be multiplied to get 532. You want to know what all the question marks in the equation below can be in order for the equation to be true:
? × ? × ? = 532
First, note that all numbers must be 532 or less, which means that there are 532 × 532 × 532 possible solutions. Thus, to get the answer to "What three numbers multiply to get 532?" we looked at 150,568,768 different combinations to see which ones equal 532.
When we looked at all those combinations, we found 54 different combinations of 3 numbers that equal 532 when multiplied together. Here are all 3 number combinations that you can multiply to get 532:
532 × 1 × 1 = 532
266 × 2 × 1 = 532
266 × 1 × 2 = 532
133 × 4 × 1 = 532
133 × 2 × 2 = 532
133 × 1 × 4 = 532
76 × 7 × 1 = 532
76 × 1 × 7 = 532
38 × 14 × 1 = 532
38 × 7 × 2 = 532
38 × 2 × 7 = 532
38 × 1 × 14 = 532
28 × 19 × 1 = 532
28 × 1 × 19 = 532
19 × 28 × 1 = 532
19 × 14 × 2 = 532
19 × 7 × 4 = 532
19 × 4 × 7 = 532
19 × 2 × 14 = 532
19 × 1 × 28 = 532
14 × 38 × 1 = 532
14 × 19 × 2 = 532
14 × 2 × 19 = 532
14 × 1 × 38 = 532
7 × 76 × 1 = 532
7 × 38 × 2 = 532
7 × 19 × 4 = 532
7 × 4 × 19 = 532
7 × 2 × 38 = 532
7 × 1 × 76 = 532
4 × 133 × 1 = 532
4 × 19 × 7 = 532
4 × 7 × 19 = 532
4 × 1 × 133 = 532
2 × 266 × 1 = 532
2 × 133 × 2 = 532
2 × 38 × 7 = 532
2 × 19 × 14 = 532
2 × 14 × 19 = 532
2 × 7 × 38 = 532
2 × 2 × 133 = 532
2 × 1 × 266 = 532
1 × 532 × 1 = 532
1 × 266 × 2 = 532
1 × 133 × 4 = 532
1 × 76 × 7 = 532
1 × 38 × 14 = 532
1 × 28 × 19 = 532
1 × 19 × 28 = 532
1 × 14 × 38 = 532
1 × 7 × 76 = 532
1 × 4 × 133 = 532
1 × 2 × 266 = 532
1 × 1 × 532 = 532
Three Numbers Multiply to Get Calculator
Do you need a different combination of three numbers that when multiplied equal a number other than 532? No problem! Please enter your number here.
What three numbers multiply to get 533?
Here is the next problem on our list that we have explained and answered for your convenience.
Copyright | Privacy Policy | Disclaimer | Contact